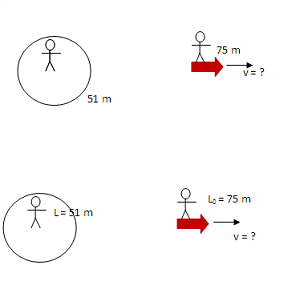
A spaceship is 75 m long according to an astronaut on the ship. As it travels away from Earth, scientists on the ground measure the length of the ship to be 51 m. How fast is the spaceship traveling away from Earth?
-
In this problem, an observer on the spaceship measures the length of the ship, and an observer on the Earth also measures the length of the ship. Any problem that relates the measurements of two observers measuring the same thing is a relativity problem.
-
How do you know which way the spaceship moves?
It doesn’t matter whether you drew the Earth on the right or the left side of the page. All that is important is that the ship moves relative to Earth in a line along the length of the ship. You only solve for speed (not direction) in this problem so all work will be the same.
In this problem, both observers are measuring the length of the spaceship. Therefore, the spaceship is the experiment or the system of interest in this problem. As always, the system of interest is identified in red.
Make sure to show all measurements on your drawing. To begin, just put each measurement down next to the observer who measured it. You will assign labels to the variables as a second step.
One of the most important things to do in Special Relativity problems is to clearly and correctly identify the reference frames of the observers. In this problem, the observers were clearly stated—an astronaut on the ship and a scientist on Earth—and so figures to represent the observers should be drawn on the ship and on the Earth.
To identify which of these observers is in the proper frame, ask what is being measured. In this case, it is the length of the spaceship and so the spaceship (and the astronaut on it) is in the proper frame. The subscript 0 on a measurement indicates it was made in the proper frame.
It doesn’t matter whether you drew the Earth on the right or the left side of the page. All that is important is that the ship moves relative to Earth in a line along the length of the ship. You only solve for speed (not direction) in this problem so all work will be the same.
-
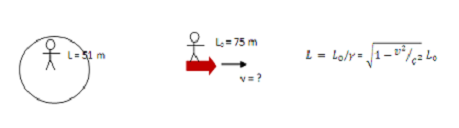
In this case, both observers measure the length of the spaceship, and so the problem is understood through the relativistic length equation regardless of what quantity is requested in the solution.
-
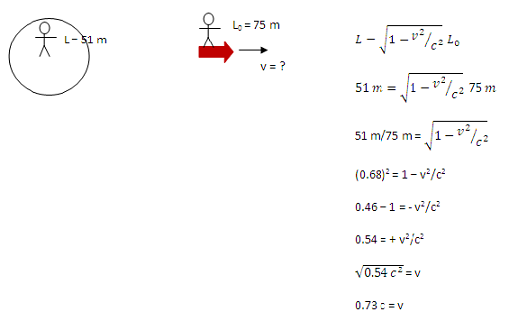
The spaceship is traveling at 73% the speed of light (or 2.2 x 108 m/s) away from Earth. No further mathematical solution is needed in this problem.
-
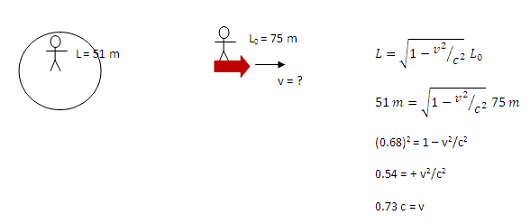
Regardless of what you were asked to find, this is a relativistic length problem because two observers in different reference frames both measure the length of the same thing. In this case, it is the spaceship whose length is measured, and so the spaceship is the proper frame (and has the longer measurement.)
Because the two measurements are noticeably different from each other, you expect that the relative speed of the frames should be a substantial fraction of the speed of light as found.
The relative speed of the two reference frames is a variable in all of the relativistic equations and so it is not a useful criterion for selecting the equation to use. You should always base your choice on the measurement that is compared by the two observers.
Why isn't the final answer in m/s?
It is convenient to solve for speeds in terms of the speed of light. If you desire an answer in terms of m/s, just substitute 3.00 x 108 m/s in for c
In this step, I divided 51 m by 75 m to get 0.68. (The units cancel.) I then squared both sides of the equation to get rid of the square root.
In this step, I not only subtracted but also multiplied both sides by -1 in order to get rid of the – sign in front of the v2 term.
In order to solve for v, I need to get it alone on one side. In this step, I multiplied each side by c2 and then took the square root of both sides.
It is convenient to solve for speeds in terms of the speed of light. If you desire an answer in terms of m/s, just substitute 3.00 x 108 m/s in for c
How can I start this problem? All of the relativity equations use velocity.
It doesn’t matter what was given or requested—you always work problems according to the key physics involved. In this case, two observers both measure the length of the spaceship, and so this is a relativistic length problem.